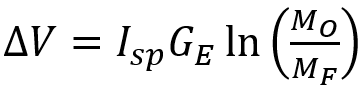Recent news in the space nerd sphere has mentioned that NASA is providing research money into investigating an oxygen pipeline, well a pipeline means that we are looking at building big stuff on the moon, and that big stuff could allow for even more big stuff to work, and provide the infrastructure this hair brained idea needs.

Previous posts on this topic looked at how varying max G loading would impact track length. Today we are trying to look at linear launch boost system as a systems engineer, aka, what are our first order boundary conditions. For those who don’t speak engineer, a first order boundary condition is something you look at to say, “hey is this idea in the right ballpark of what physics says is possible” As an example, if someone says that their electric SUV can go 1000 km on a battery the size of a walnut. You can do the math on how much energy a walnut sized battery can contain and, at least with current chemical energy options you are likely to say that the statement is false* and know you can ignore those claims.

When engineers are working on a project they want to be able to know what is the minimum needed to do a thing and what limitations are on the maximum of doing that thing.

In the first posts on air hockey on the moon we looked at how different rates of acceleration and track shape would impact our ability to launch stuff off the lunar surface. There our key take aways were, higher acceleration on the trackway reduces how much trackway you need, and a straighter track reduces peak g loading. That’s a good starting point, but we need to get deeper into the weeds if we are going to get a real sense of how “effective” a linear trackway would be.

If no major boundaries were imposed we would want a track of an arbitrarily long length, 100+ km and be able to accelerate any payload to an orbital velocity, 2380 m/s.

Some questions we will look at today.

What rate of acceleration is too high?

Is there a sweet spot for track length?

If we can’t get to orbital velocity using just our accelerator track what kind of fuel savings will we see?

How does the type of fuel our spacecraft uses impact any potential fuel savings? (originally I wrote “does the type of fuel” ignoring the word how, but for those technically minded folks who might read this I didn’t want the answer for them to immediately be a “duh yes”)

Peak acceleration is both tough and straightforward at the same time. The straightforward answer is, if the trackway is designed for people you want to keep net G forces on crew members relatively safe just shy of 4 Gs is the peak seen by the command module of Apollo 11 realistically you’ll want lower than that as astronauts who have been on the lunar surface for a long time may not be able to safely handle those 4 Gees. An arbitrary peak acceleration of 2.5 Gees is what I’m going to use for many calculations as it makes my life easier. Now this peak acceleration is intended for crewed launches, for uncrewed launches, that number is adjacent to being arbitrary. The reason I say adjacent to arbitrary is that our linear trackway will need to be designed to push some kind of preferred payload, in this case I am going to assume that the “preferred payload” is crewed vehicles. So whatever amount of force we need to launch our crewed vehicles is likely to be a little bit lower than our peak payload.

For sake of convenience and based on a whim, for future reference our crewed lunar transporter will weigh in at about 2x that of the Apollo Lunar lander, which massed in at 15,103kg. Our spacecraft will mass at 30,000 kg.

Now that we have our peak acceleration of 2.5 Gees we can start to look at how track length impacts our launch velocity requirements.

OK, we see that as we keep increasing track length we keep getting faster, shocker, but at what point do our gains seem less useful, for that let’s zoom in at track lengths under 10 km and make the vertical axis log scale to make things more obvious.

Now things look a bit clearer, after accelerating for 800 meters our spacecraft has shaved 200 m/s of delta V needed to get into orbit. To shave an additional 200 m/s off our delta V requirements we will need 3275 m of trackway (that’s going from shy of half of a mile of track to 2 miles of trackway. Basically, every time you want to double your velocity you need to quadruple your track length.

Next, we want to look at how the fuel efficiency of our spacecraft impacts how much mass our spacecraft saves. We are entering the rocket science zone. When you are doing a basic estimation of how big a spacecraft will need to be to get your mission done you use the rocket equation

The Rocket Equation showing deltaV= ISP* G * ln(MO/.MF)

This equation boils down the question of getting around space as simply as you realistically can. To get from point A to point B in orbital mechanics you need a certain amount of delta V, or change of velocity. Knowing how much delta V you need you then need to have an idea of how efficient your fuel is, that’s the specific impulse, which is generally measured in seconds, more seconds is more better. The variable represents the acceleration of gravity here on Earth 9.81 m/s^2, this turns the specific impulse into a velocity value. (Why don’t we just measure ISP in m/s TRADITION, and while the origins were explained to me I can’t remember right now and hey, this is already a wall of text). Our final variables and represent the mass of our spacecraft at the beginning of changing direction and the mass of the spacecraft at the end of the burn respectively.

What this chart is trying to convey is how much mass our spacecraft will save based on a combination of track length and ISP, assuming an average acceleration on the track of 2. 5G. **

Unsurprisingly, at least for people who spend their time thinking about this stuff, the more efficient your rocket ship’s engines/fuel combination, the less mass you save by getting this initial boost. For our super inefficient engines with an ISP=50s, it takes less than 250m of being boosted on our track to shave 20% off our launch mass, for ISP=100s it takes almost a kilometer of trackway, at ISP=350s we are looking at 12 km of track, that’s massive.

This highlights some critical questions that a human rated crew launch system would need to be able to answer. If we are only launching people a few times a year on a reusable spacecraft, it is likely cheaper to look for relatively rare water to power an efficient hydrogen oxygen burning spacecraft. Where this idea is still worth exploring is for accelerating payloads much faster than our initial 2.5 Gees. At 5 Gees we see modest improvements where our ISP 450 spacecraft would save about 10% of its launch weight, in theory. We must add the qualifier “in theory” as the rocket equation doesn’t describe the physical design of a spacecraft, aside from how much mass changes for a particular delta V. A mechanical design that works at one rate of acceleration may not be strong enough, and so additional design work would need to be done. More likely that redesign would end up having heavier structural elements, those heavier structural elements could easily reduce our mass savings.

At this time it appears to me that the Lunar Air Hockey Table is one of those, cool ideas with mixed utility in the real world. In a future scenario where Lunar outposts want to launch a wide array of payloads into orbit at such volumes that using locally available water isn’t practical and “weirder” fuels like potassium, aluminum, sulfur, etc… are being used for rocket boosters, then the trackway could make sense. For a future where the moon is primarily exporting solid slugs of refined metals, a gun solution is more practical.

Hopefully this was interesting, or at least not painful to read, please reach out with questions if you have any.

** a note on the methodology for mass savings, for each ISP I had the worksheet calculate the mass ratio our spacecraft would need to have to 2380 m/s with no boost, R basic, for basic mass ratio. Then after calculating the reduction of mass ratio requirements for our boosted spacecraft, R boosted. To determine the percentage of mass savings I used the equation (1-(Rboosted/R_basic))*100%. Hopefully, this explanation makes sense (if I am obviously wrong please feel free to let me know, solo projects have a nasty habit of allowing simple oversights)

Thumbnail Image courtesy of wikimedia

Description

English: Liquid oxygen

Tiếng Việt: Oxy ở dạng lỏng (O3)

Date10 October 2010, 13:27:58Sourcehttp://www.afcent.af.mil/Units/455thAirExpeditionaryWing/Photos/tabid/5491/igphoto/2000316697/Default.aspxAuthorStaff Sgt. Nika Glover, U.S. Air Force

Air Hockey On the Moon 2023